RECREATIONS OF A PHILOSOPHER
| "C |
HARLES BABBAGE, Esq., M.A.," as he calls himself, is known to scientific men as the author of the Calculating Machine, one of the most complicated and ingenious contrivances of human invention for lightening the burdens of the memory and the intellect. He is better known to readers of English newspapers as the persistent opponent of street music, and persecutor of organ-grinders and their monkeys.
Like some other philosophers Mr. Babbage has a high opinion of his own merits, and a poor opinion of most of his contemporary lovers of wisdom. Nevertheless, he is not above amusing the world as well as instructing it; and in a recently published volume he takes the public into his confidence, and tells it some pleasant stories concerning the pursuits and mishaps of a philosopher in this nineteenth century.
He begins by telling us that he has had frequent requests from printers for his life, and on various terms; some offering to pay him for the autobiographic volume, others willing to be paid for printing it, and yet others ready to print without pay to either the philosophic author and subject or to themselves. One ingenious publisher made him a distinct offer to print what and as much as he chose for tenpence a line. It does not appear that his offer was accepted.
Mr. Babbage is in doubt about his origin and remote ancestry. He flattered himself, indeed, that he was descended from Tubal-Cain, who was, like himself, an ingenious worker in iron; but the reminder of a friend that Tubal-Cain also invented the organ put to naught this hypothesis -- for Mr. Babble will allow no kinship with the patron of the organ-grinding craft.
He tells us that in his childhood he was noted for a passion to examine the inside of his toys, which commonly resulted in their destruction. Being sent to boarding-school at the age of five, he was troubled with doubts as to the existence of a devil, and, to solve these, made an attempt to raise the devil by the orthodox and well-known way of saying the Lord's Prayer backward. He previously questioned his fellow-scholars closely as to the different shapes in which the Prince of Darkness was known to have presented himself, and learned that he had appeared as a rabbit, an owl, a black cat very frequently, a raven, and a man with cloven foot--the last commonly. His experiment, made in a garret, was unsuccessful, and therefore unsatisfactory; and still in doubt upon this point of faith, he determined to decide it by an appeal to chance. If he found a certain door open he would believe the Bible; if not, he would disbelieve. Unfortunately Mr. Babbage does not remember the result.
Later, at another school, he had a curious trial of pertinacity with Frederic Marryatt, afterward sea-captain and novelist. Young Babbage and some other boys undertook secretly to rise every morning at three, and silently slip to the school-room, there to study till half past five. Marryatt wanted to join this singular company, but was denied. He placed his bed across the edge of the door, in order to be wakened by Babbage when he went out, but this was softly removed; he tied twine to the door and to his foot, but this was cut; he fastened a chain to the door, but his antagonist, after a defeat for a single night, procured a pair of pliers and unbent one of the links; then Merryatt made all fast with a stout chain and padlock, too strong for the other's efforts and instruments, and thereupon Babbage "changed his base," to use a term sadly familiar to us Americans; he tied a string to the chain, and when Marryatt fell asleep gave it a pull; up jumped the latter, but found no one near the door. His opponent repeated this trick several times during the night, and succeeded in annoying Marryatt, but not in getting out. At last a compromise was effected; the future novelist became a member of the three o'clock club, but turned it into a frolic and banished study.
While at Cambridge the embryo philosopher undertook to write a grammar and dictionary for a universal language, but gave it up. In his studies of mathematics he found the system of notation of Leibnitz far better than the system in use at Cambridge, and held private meetings to encourage the substitution of d's for dots. The society he thus formed undertook to print an essay, for which Mr. Babbage cleverly proposed a title, which at the same time hit off some of the theological disputes then raging. He suggested that the essay should be called, "The Principles of pure D-ism, in opposition to the Dot-age of the University."
Among the university clubs at that time was a "Ghost Club," which saw no ghosts; and another called the "Extractors," which had the following rules:
1st. Every member shall communicate his address to the Secretary once in six months.
2d. If this communication is delayed beyond twelve months, it shall be taken for granted that his relatives had shut him up as insane.
3d. Every effort, legal and illegal, shall be made to get him out of the mad-house. Hence the name of the club--The Extractors.
4th. Every candidate for admission as a member shall produce six certificates--three that he is sane, and three others that he is insane.
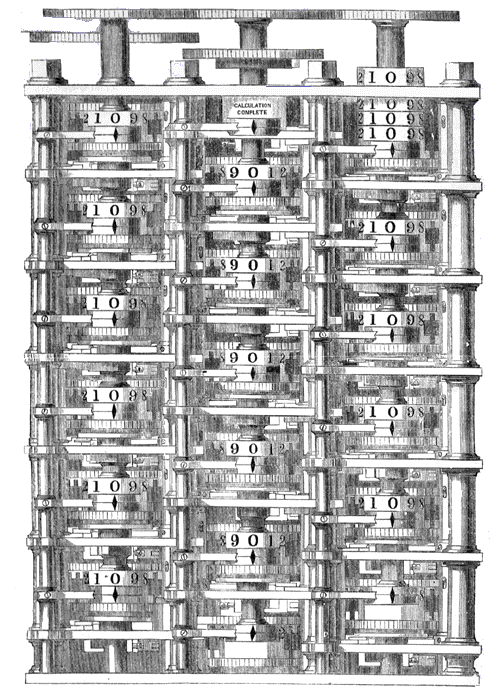
We come now to the great work of Mr. Babbage, that has employed him for over forty years. and which still engages his attention--The Calculating or Difference Engine. The idea of contriving a machine which should perform the drudgery of mathematical and arithmetical calculations first occurred to him in 1812, at Cambridge. In 1822 he completed his first machine. In 1823 he began a large one for the British Government, a representation of the finished portion of which is shown on the previous page. This prints its results, so that all errors, even those of the compositor or type-setter, are avoided.
What, then, is a Calculating or Difference Machine? the reader asks. Perhaps a brief history of Mr. Babbage's invention will convoy to the general reader the clearest and most interesting notion of this wonderful conglomeration of wheels, which does the drudgery of a hundred first-rate mathematicians without tiring. and without a possibility of error.
Mr. Babbage relates that he was one day sitting in the rooms of the Analytical Society at Cambridge, with his head leaned forward upon the table, musing; a friend who entered asked, "What are you dreaming about?" to which the philosopher replied, "I am thinking that all these tables of logarithms might be calculated by machinery." Now the calculating machine. the fruit of this thought, does--being moved by the turning of a crank--calculate tables of logarithms, and other tables necessary to the labors of all devoted to the higher branches of mathematics. Feed it with figures, set it properly, and it will turn out the required results with unfailing accuracy and with great speed; and not only that--it prints these results on a prepared surface, from which stereotype casts can be and are taken for the use of the printer. But it does more even than this; for it actually performs the wonderful feat of correcting any error of the attendants whose duty it is to feed this intelligent creature with the crude figures which it is to work up. If he inserts the wrong numbers it rejects them, for it will not calculate an impossible or absurd problem.
Mr. Babbage tells us that he did not think it useful to construct a machine which should execute only the mere isolated operations of arithmetic. Such a machine would be too limited in its powers. But "the method of differences supplied a general principle by which all tables might be computed, through limited intervals, by one uniform process;" and this method required the use of mechanism for addition only. The "method of differences" may be briefly explained to consist in forming tables of results, by means of successive additions of the constant term of difference. Thus, suppose it were required to be known what is the value of any number of pounds of meat, from 1 to 100, at five cents per pound; the shortest way for a school-boy would be to multiply the pounds by five, and set down the result. But the mathematician tells him that by this process, which secures each time an independent result, there is no possibility of checking error in the completed table except by revising separately each of its hundred parts; whereas, if the table had been formed by successive additions of five, the constant difference, an examination of the last term would have at once proved the correctness of the whole table. "But that is so slow!" Therefore, said Mr. Babbage, let us perform these additions by machinery.
Now, to take a more intricate example, let any boy who reads this lay down five marbles separately in a row. Then let him place two marbles under each one but the first; and next three more under each group, beginning with the third; and again four more under each, beginning with the fourth; and so on, commencing always one group later, and adding one marble more each time. He would have such an arrangement as this:
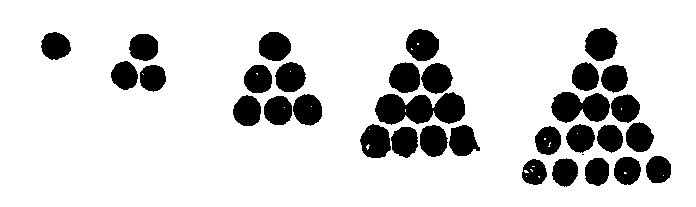
Now he could with a, little trouble count the marbles in each group; but suppose he should desire to know how many marbles would be contained, not in the seventh of such an ascending series, which he has, but in the thirtieth, say, how is he to discover this? If he will analyze his groups he will see that they are composed by additions made after a certain steady and regular scale. Thus his table will stand:
| Table. | 1nd Difference. | 2nd Difference. | |
| Number of the Group. | Number of Marbles in each Group. | Difference between the number of Marbles in each Group and that in the next. | |
| 1 | 1 | 1 | 1 |
| 2 | 3 | 2 | 1 |
| 3 | 6 | 3 | 1 |
| 4 | 10 | 4 | 1 |
| 5 | 15 | 5 | 1 |
| 6 | 21 | 6 | |
| 7 | 28 | 7 |
Now this table can be calculated, like that concerning butcher's meat, in two ways--by mere addition, or by a method deducible from the elements shown in the table, by which each result would be obtained independently. Thus, if you desire the number of marbles or units in the fifth group,
Take the number of the group..... 5 Add 1 to this ................... 6 Multiply these.................. 30 Divide by 2..................... 15
and you have the number of marbles in the fifth group.
If, now, the boy asks, "What is the use of such tables?" Mr. Babbage replies that they are of great use--the very Table about which we have been reasoning possesses a special name--it is called a Table of Triangular Numbers. Almost every general collection of Tables hitherto published contains portions of it of more or less extent. Above a century ago a volume in small quarto, containing the first 20,000 triangular numbers, was published at the Hague by E. De Joncourt, A.M., and Professor of Philosophy. I can not resist quoting the author's enthusiastic expression of the happiness he enjoyed in composing his celebrated work:
"The Trigonals here to be found, and nowhere else, are exactly elaborate. Let the candid reader make the best of these numbers, and feel (if possible) in perusing my work the pleasure I had in composing it. That sweet joy may arise from such contemplations can not be denied. Numbers and lines have many charms unseen by vulgar eyes, and only discovered to the unwearied and respectful sons of Art. In features the serpentine line (who starts not at the name) produces beauty and love; and in numbers high powers and humble roots give soft delight. Lo! the raptured arithmetician! Easily satisfied, he asks no Brussels lace, nor a coach and six. To calculate contents his liveliest desires, and obedient numbers are within his reach."
It may now be stated that mathematicians have discovered that all the tables most important for practical purposes, such as those relating to Astronomy and Navigation, can, although they may not possess any constant differences, still be calculated in detached portions by that method. Hence the importance of having machinery to calculate by differences, which, if well made, can not err; and which, if carelessly set, presents in the last term it calculates the power of verification of every antecedent term.
We find, then, in the first place, that the calculating machine or Difference Engine of Mr. Babbage performs its useful labor so far by the process of addition. Now he remarks that if it requires a certain number of seconds for a man to add numbers, as
82456
77777
But by properly-arranged machinery these five separate processes could be carried on simultaneously; and it would make no difference in the time required if there were a dozen places instead of five. So much is gained, then, in point of speed.
But suppose there is "one to carry?" Suppose you wanted to add
How are you going to teach the machine to remember? And not only to remember what and where to "carry," but to make it perform this office. Mr. Babbage says:
"The mechanical means I employed to make these carriages bear some slight analogy to the operation of the faculty of memory. A toothed wheel had the ten digits marked upon its edge: between the nine and the zero a projecting tooth was placed. Whenever any wheel, in receiving addition, passed from nine to zero, the projecting tooth pushed over a, certain lever. Thus, as soon as the nine seconds of time required for addition were ended, every carriage which had become due was indicated by the altered position of its lever. An arm now went round, which was so contrived that the act of replacing that lever caused the carriage which its position indicated to be made to the next figure above. But this figure might be a nine, in which case, in passing to zero, it would. put over its lever, and so on. By placing the arms spirally round an axis these successive carriages were accomplished.
"Multitudes of contrivances were designed, and almost endless drawings made, for the purpose of economizing the time and simplifying the mechanism of carriage. In that portion of the Difference Engine in the Exhibition of 1862 the time of carriage has been reduced to about one-fourth part of what was at first required.
"At last having exhausted, during years of labor, the principle of successive carriages, it occurred to me that it might be possible to teach mechanism to accomplish another mental process, namely--to foresee. This idea occurred to me in October, 1834. It cost me much thought, but the principle was arrived at in a short time. As soon as that was attained, the next step was to teach the mechanism which could foresee to act upon that foresight. This was not so difficult: certain mechanical means were soon devised which, although very far from simple, were yet sufficient to demonstrate the possibility of constructing such machinery; The process of simplifying this form of carriage occupied me, at intervals, during a long series of years."
If now the reader will turn to the engraving of Mr. Babbage's machine he will notice three columns, each containing six wheels, each wheel having upon its face the ten digits. The lower wheel represents units, the next tens, and so on, the last of course standing in each column for tens of thousands. On the right hand column the table to be calculated by the machine is expressed. It will of course receive or express any number up to 99999. The second column is the first difference column, a title which will be comprehended by reference to the marble problem. The third column is the second difference column; by these two are expressed and calculated the differences.
Now, Mr. Babbage tells us:
"The mechanism is so contrived that whatever may be the numbers placed respectively on the figure-wheels of each of the three columns, the following succession of operations will take place as long as the handle is moved:
"1st. Whatever number is found upon the column of first differences will be added to the number found upon the table column.
"2d. The same first difference remaining upon its own column, the number found upon the column of second differences will be added to that first difference.
"It appears, therefore, that with this small portion of the engine any table may be computed by the method of differences, provided neither the table itself, nor its first and second differences, exceed five places of figures.
"If the whole engine had been completed it would have had six orders of differences, each of twenty places of figures, while the three first columns would each have had half a dozen additional figures."
Our philosopher adds: "On two occasions I have been asked, 'Pray, Mr. Babbage, if you put into the machine wrong figures, will the right answers come out?' I am not able rightly to apprehend the kind of confusion of ideas that could provoke such a question. I did, however, explain the following property, which might in some measure approach toward an answer to it. It is possible to construct the Analytical Engine in such a manner that after the question is once communicated to the engine it may be stopped at any turn of the handle, and set on again as often as may be desired. At each stoppage every figure-wheel throughout the engine which is capable of being moved without breaking may be moved on to any other digit. Yet after each of these apparent falsifications the engine will be found to make the next calculation with perfect truth. The explanation is very simple, and the property itself useless."
Mr. Babbage is now engaged upon the construction of an "Analytical Engine"--a larger calculating machine, of the most various and general powers. For this he has so perfected the system of carrying tens in addition that he has reduced the actual time required for the addition of two numbers of any number of digits to nine units of time for the addition and one for the carriage. By its help the following astounding results are attained in the simpler operations of arithmetic:
Sixty additions or subtractions may be completed and printed in one minute.
One multiplication of two numbers, each of fifty figures, in one minute.
One division of a number having one hundred places of figures by another of fifty in one minute.
We have already mentioned that the machine calls for a table of numbers when it needs it in the process of a calculation, and refuses to accept the wrong table at the hands of the attendant. There is here an apparent choice of means, a power to distinguish between right and wrong which seems almost human. But not quite, as the following curious incident will demonstrate. Mr. Babbage amused himself by examining into the plan upon which an automaton might be constructed to play a game of skill, such as chess. He satisfied himself that any such game might be played by an automaton. Having got so far, he thought of constructing an automaton who should play at the boys' and girls' game of tit-tat-to. He found it easy to calculate all the possible combinations of this simple game; and he sketched the means by which his machine-man should be properly guided. He readily provided beforehand by calculation for every possible move, because each followed necessarily another--except in the case where, of two possible moves, neither was actually preferable, but each was equally inducive to winning the game. "In this case no reason existed within the machine to direct its choice," and unless some provision were made the machine would attempt to make two contradictory motions!
In connection with the Calculating Machine, Mr. Babbage has an interesting reminiscence of the Duke of Wellington: "One morning the Duke called in Dorset Street with the late Countess of Wilton, to whom he wished me to show the Difference Engine. Its home was at that period in my drawing-room. We sat round it while I explained its mode of action and made it calculate some small table of numbers. When I had concluded my explanation, Lady Wilton, addressing me, said, 'Now, Mr. Babbage, can you tell me what was your greatest difficulty in contriving this machine?' I had never previously asked myself that question; but I knew the nature of it well. It arose not from the difficulty of contriving mechanism to execute each individual movement, for I had contrived very many different modes of executing each; but it really arose from the almost innumerable combinations among all these contrivances--a number so vast that no human mind could examine them all. It instantly occurred to me that a similar difficulty must present itself to a general commanding a vast army when about to engage in a convict with another army of equal or of greater amount. I therefore thought it must have been felt by the Duke of Wellington, and I determined to make a kind of psychological experiment upon him. Carefully abstaining from any military term, I commenced my explanation to Lady Wilton. I soon perceived by his countenance that the Duke was already in imagination again in Spain. I then went on boldly with the explanation of my own mechanical difficulty; and when I had concluded the Duke turned to Lady, Wilton and said, 'I know that difficulty well.'"
At a dinner party the characters of the French marshals became the subject of conversation. The Duke, being appealed to, pointed out freely their various qualities, and assigned to each his peculiar excellence. One question, the most highly interesting of all, naturally presented itself. One of the party, addressing the Duke, said: "Well, Sir, how was it that, with such various great qualities, you whipped them all, one after another?"
The Duke was evidently taken by surprise. He paused for a moment or two, and then said: "Well, I don't know exactly how it was; but 1 think, that if any unexpected circumstance occurred in the midst of a battle, which deranged its whole plan, I could perhaps organize another plan more quickly than most of them."
The poet Rogers was another friend of our philosopher. Once, at a large dinner party, Mr. Rogers was speaking of an inconvenience arising from the custom, then commencing, of having windows formed of one large sheet of plate-glass. He said that a short time ago he sat at dinner with his back to one of these single panes of plate-glass: it appeared to him that the window was wide open, and such was the force of imagination that he actually caught cold! Mr. Babbage neatly capped this story by an experience of his own. Being in the country, and without a night-cap, he feared to catch cold, and it occurred to him to tie a, string over his head under his chin. This device satisfied his imagination or habit, and he slept as comfortably as though his head had been enveloped in the customary night-cap.
He knew, also, Vidocq, the celebrated French thief-taker, who, it seems, had a, remarkable power of altering his height, which must have been the envy of many of his victims. He could make himself, by some curious contortion of his body, appear to be about an inch and a half shorter than he really was; He found this faculty very useful as a disguise. Mr. Babbage records that Vidocq was not at all an adept in picking locks.
The recreations of a philosopher naturally
partake of his general cast of mind. Thus we
are not surprised to read that Mr. Babbage
nearly drowned himself in trying a contrivance.
of his own for walking on the water; that he
suffered himself to be baked in an oven; that
he is an adept in picking locks; and that he
delights in the abstruse art of deciphering, which
is practiced occasionally in these days by the
staff officers of some of our generals when an
enemy's dispatch in cipher falls into their hands.
While on this last subject he mentions an amusement
called squaring words, which we recommend
as a pretty winter-evening game for the
parlor or the country fireside. It is thus practiced:
Let the given worst to be squared be
Dean. It is to be written horizontally and also
vertically, thus:
| d | e | a | n |
| e | . | . | . |
| a | . | . | . |
| n | . | . | . |
And it is required to fill up the blanks with such letters that each vertical column shall be the same as its corresponding horizontal column, thus:
| d | e | a | n |
| e | a | s | e |
| a | s | k | s |
| n | e | s | t |
Nor are we surprised. that when this philosopher has a nuisance to contend with he makes a systematic statement of his annoyance. It is well known that Mr. Babbage has been for some years waging war with the itinerant street musicians who abound in London. He gives the following curious list of what he calls the "instruments of torture permitted by the Government to he in daily and nightly use in the streets of London:" Organs, brass bands, fiddles, harps, harpsichords, hurdy-gurdies, flageolets, drums, bagpipes, accordions, half-penny whistles, tom-toms, trumpets, and the human voice in various forms. The performers he classifies too:
Musicians. Instruments. Italians....................... Organs. Germans ....................... Brass bands. Natives of India............... Tom-toms. English ....................... Brass bands, fiddles, etc. The lowest class of clubs...... Bands with double drum.
He asserts that during the last twelve years one-fourth of his working power has been destroyed by these street nuisances. This is our philosopher's pet grievance.